北航微分几何讨论班(2021年春第7讲)
题目:Sharp affine isoperimetric inequalities for the volume decomposition functionals of polytopes
报告人:熊 革 教授(同济大学)
报告时间:2021.4.30 10:00-11:00
腾讯会议号:570 714 666
摘要:In this talk, I will introduce our very recent work on the geometry of polytopes. We prove that the nth power of the volume functional  of polytopes P in
of polytopes P in 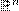 ,
,
according to dimensions of the spaces spanned by any n outer normal unit vectors of P, is naturally decomposed into n homogeneous polynomials with degree n. Several new sharp affine isoperimetric inequalities for these functionals are established, which essentially characterize the geometric and algebraic structures of polytopes.
报告人简介:熊革,同济大学教授、博士生导师。主要研究凸体几何。他解决了Lp 静电容量的Minkowski 问题;提出并证明了“Lp transference principle”,对Lp Brunn-Minkowski型不等式进行了统一处理。他解决了凸体几何中的几个公开问题,包括锥体积泛函仿射极值的Lutwak-Yang-Zhang公开问题的2,3维情形;由截面确定凸体的Baker-Larman公开问题的2维情形;完全解决了G. Zhang关于凸体的John 椭球与对偶惯性椭球的一致性问题。
他在国际纯数学重要期刊如JDG, AIM, JFA, CVPDE, IUMJ, IMRN, CAG, DCG等发表论文近30篇。他的研究成果或被写入凸体几何的经典教材中、或被发表在国际顶尖期刊上的文章多次引用和正面评价。
邀请人:张世金
